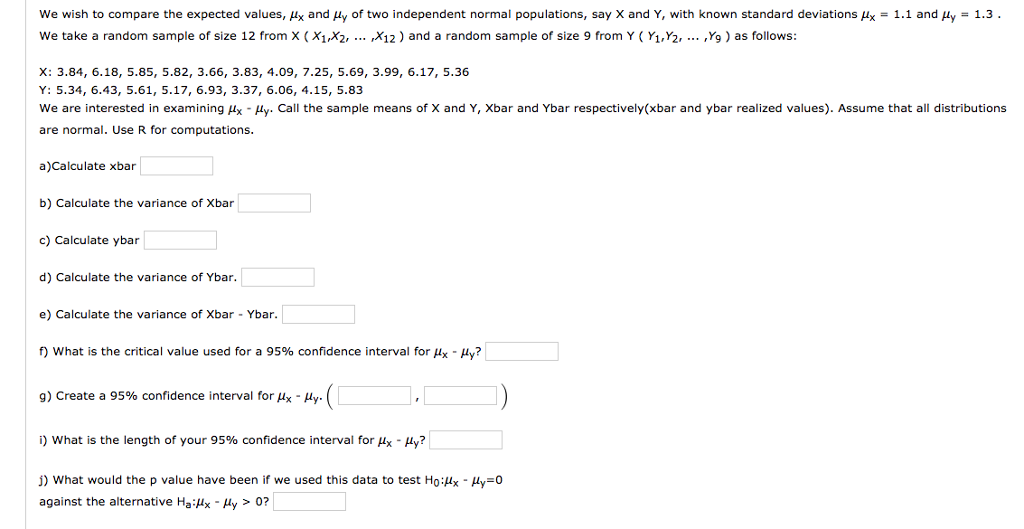
Show transcribed image text We wish to compare the expected values, Hx and phy of two independent normal populations, say X and Y, with known standard deviations = 1.1 and phy = 1.3. we take a random sample of size 12 from X (X1,X2, … ,X12) and a random sample of size 9 from Y (Y1,Y2, … ,Yg) as follows: X: 3.84, 6.18, 5.85, 5.82, 3.66, 3.83, 4.09, 7.25, 5.69, 3.99, 6.17, 5.36 Y: 5.34, 6.43, 5.61, 5.17, 6.93, 3.37, 6.06, 4.15, 5.83 We are interested in examining 4x – Hy. Call the sample means of X and Y, Xbar and Ybar respectively(xbar and ybar realized values). Assume that all distributions are normal. Use R for computations. a)Calculate xbar || b) Calculate the variance of Xbar c) Calculate ybar| d) Calculate the variance of Ybar. e) Calculate the variance of Xbar – Ybar. f) What is the critical value used for a 95% confidence interval for Ax – hy? 9) Create a 95% confidence interval for dx – ly. ( , 1) What is the length of your 95% confidence interval for pux – hy? 3) What would the p value have been if we used this data to test Houx – Hy=0 against the alternative Ha:Ax – Hy > 0?
We wish to compare the expected values, Hx and phy of two independent normal populations, say X and Y, with known standard deviations = 1.1 and phy = 1.3. we take a random sample of size 12 from X (X1,X2, … ,X12) and a random sample of size 9 from Y (Y1,Y2, … ,Yg) as follows: X: 3.84, 6.18, 5.85, 5.82, 3.66, 3.83, 4.09, 7.25, 5.69, 3.99, 6.17, 5.36 Y: 5.34, 6.43, 5.61, 5.17, 6.93, 3.37, 6.06, 4.15, 5.83 We are interested in examining 4x – Hy. Call the sample means of X and Y, Xbar and Ybar respectively(xbar and ybar realized values). Assume that all distributions are normal. Use R for computations. a)Calculate xbar || b) Calculate the variance of Xbar c) Calculate ybar| d) Calculate the variance of Ybar. e) Calculate the variance of Xbar – Ybar. f) What is the critical value used for a 95% confidence interval for Ax – hy? 9) Create a 95% confidence interval for dx – ly. ( , 1) What is the length of your 95% confidence interval for pux – hy? 3) What would the p value have been if we used this data to test Houx – Hy=0 against the alternative Ha:Ax – Hy > 0?







